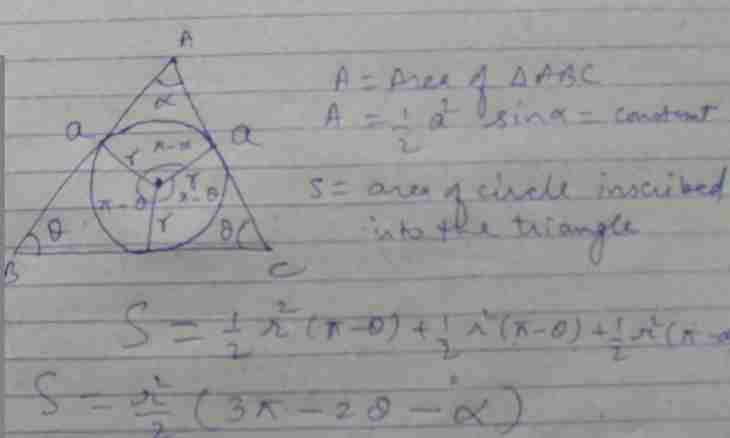The circle can be entered in a corner or in a convex polygon. In the first case it concerns both sides of angle, in the second — all parties of a polygon. Position of its center in both cases is calculated in the similar ways. It is necessary to carry out additional geometrical constructions.
It is required to you
- - polygon;
- - corner of given size;
- - a circle with the set radius;
- - compasses;
- - ruler;
- - pencil;
- - calculator.
Instruction
1. To find the center of an inscribed circle means to define its situation concerning top of a single corner or corners of a polygon. Remember where there is a center of the circle entered in a corner. It lies on a bisector. Construct a corner of given size and halve it. You know the radius of an inscribed circle. At an inscribed circle it is also the shortest distance from the center to a tangent, that is a perpendicular. In this case the side of angle is tangent. Construct the perpendicular equal to the set radius to one of the parties. Its final point has to be on a bisector. At you the rectangular triangle turned out. Call it, for example, the WASP. About is a top of a triangle and at the same time the center of a circle, OS — radius, and OA — a bisector piece. The corner of OAS is equal to a half of an initial corner. According to the theorem of sine find a piece of OA which is a hypotenuse.
2. For positioning of the center of an inscribed circle in a polygon execute similar constructions. The parties of any polygon by definition are tangents to an inscribed circle. Respectively, the radius which is carried out to any point of contact will be perpendicular to it. In a triangle the center of an inscribed circle is a point of intersection of bisectors, that is its distance from corners is defined just as in the previous case.
3. The circle entered in a polygon at the same time is entered and in each its corner. It follows from its definition. Respectively, the distance of the center from each of tops can be calculated just as in a case with a single corner. It is especially important to remember it if you deal with the wrong polygon. At calculations of a rhombus or square it is enough to carry out diagonals. The center will coincide with a point of their crossing. It is possible to determine its distance from square tops by Pythagorean theorem. In a case with a rhombus the theorem of sine or cosines works, depending on what corner you use for calculations.