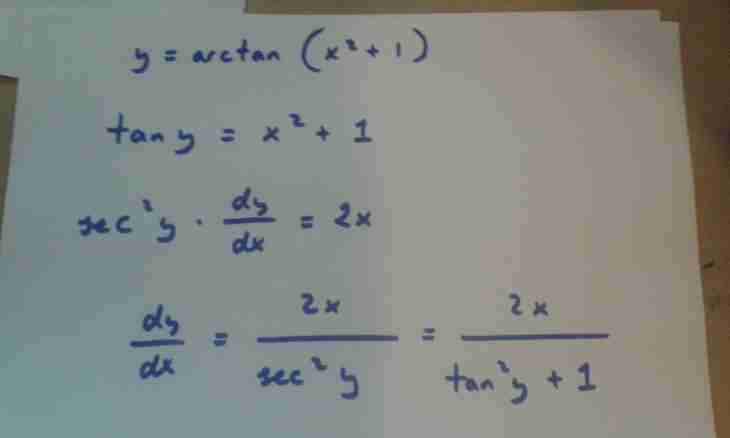Emergence of differential calculus is caused by need to solve specific physical objectives. It is supposed that the person owning differential calculus is able to take derivative of various functions. Can you take derivative of the function expressed by fraction?
Instruction
1. Any fraction has numerator and a denominator. In the course of finding of a derivative from fraction it will be required to find separately derivative numerator and a denominator derivative.
2. To find a derivative from fraction, a numerator derivative to a domnozhta on a denominator. Subtract the denominator derivative multiplied by numerator from the received expression. Divide result into a denominator in a square.
3. Example of 1 [sin (x)/cos (x)]’ = [sin’ (x) · cos (x) — cos’ (x) · sin (x)] / cos? (x) = [cos (x) · cos (x) + sin (x) · sin (x)] / cos? (x) = [cos? (x) + sin? (x)] / cos? (x) = 1 / cos? (x).
4. The received result is nothing else as tabular value of derivative function of a tangent. It also is clear, the sine relation to a cosine and is, by definition, a tangent. So, tg (x) = [sin (x)/cos (x)]' = 1/cos? (x).
5. Example 2 [(x? — 1) / 6x]’ = [(2x · 6x — 6 · x?) / 6?] = [12x? — 6x?] / 36 = 6x? / 36 = x? / 6.
6. A special case of fraction is such fraction which has in a denominator unit. It is simpler to find a derivative from fraction of a such type: it is enough to present of it in the denominator form with degree (-1).
7. Example (1 / x)' = [x^(-1)]' =-1 · x^(-2) =-1/x?.